Video:
Take our online poll:
AI Analysis:
Where the percent increase appears more significant when prices rise compared to when prices fall, is due to the nature of percentage changes and the concept of relative change.
When prices rise:
Starting Point: Let's say you start with a lower price, and it increases. For example, if a product costs $50 and then increases to $60, the percentage increase is (60 - 50) / 50 = 20%.
Relative to a Smaller Base: In this case, the percentage increase is relative to the initial, lower price ($50). Since you're dividing the change by a smaller base, the percentage increase appears larger.
When prices fall:
Starting Point: Conversely, if prices fall, you're starting with a higher price. For example, if a product costs $60 and then decreases to $50, the percentage decrease is (60 - 50) / 60 = 16.67%.
Relative to a Larger Base: The percentage decrease is relative to the initial, higher price ($60). When you divide the change by a larger base, the percentage decrease appears smaller in magnitude.
This phenomenon is a result of the way percentages work. The same numerical change in price can result in different percentage changes depending on the reference point. When prices rise, it seems like a bigger percentage increase because it's relative to a smaller starting price, while a price decrease appears as a smaller percentage decrease because it's relative to a higher starting price.
This difference is important to understand when interpreting economic data or financial news because it can affect people's perceptions of price changes and their impact on their budget or investments.
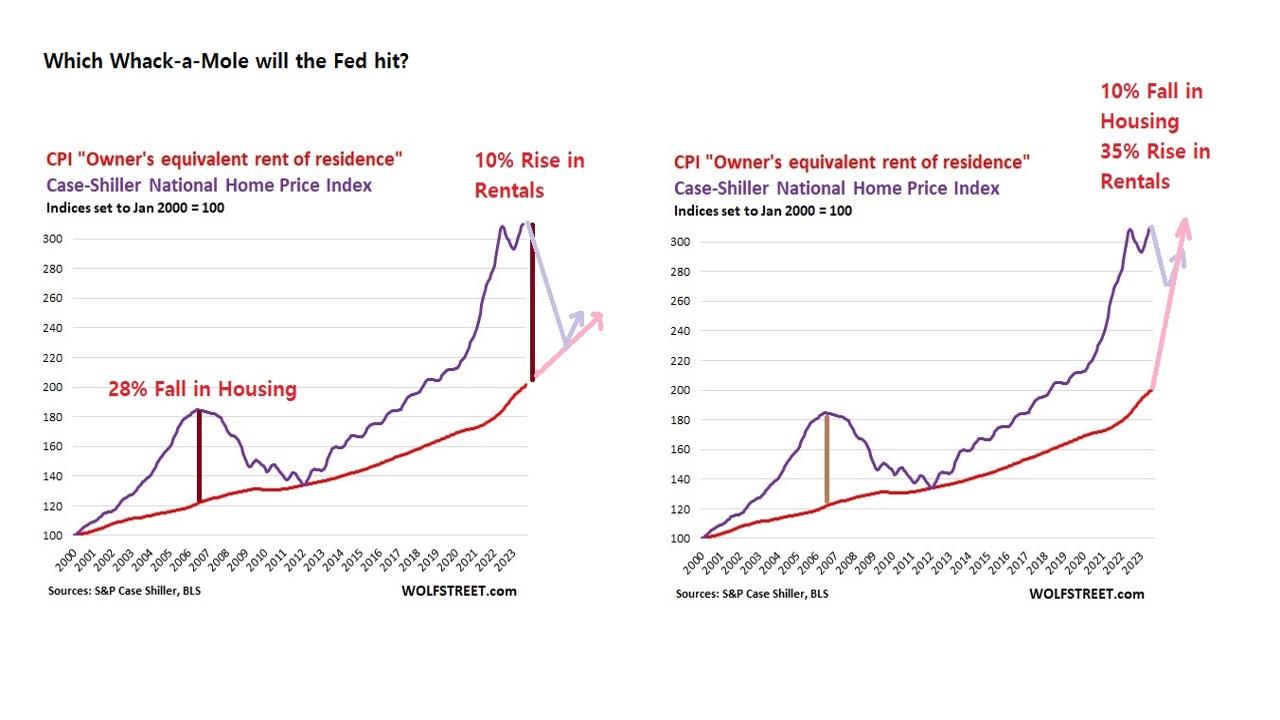
Chart:
References:


Comments